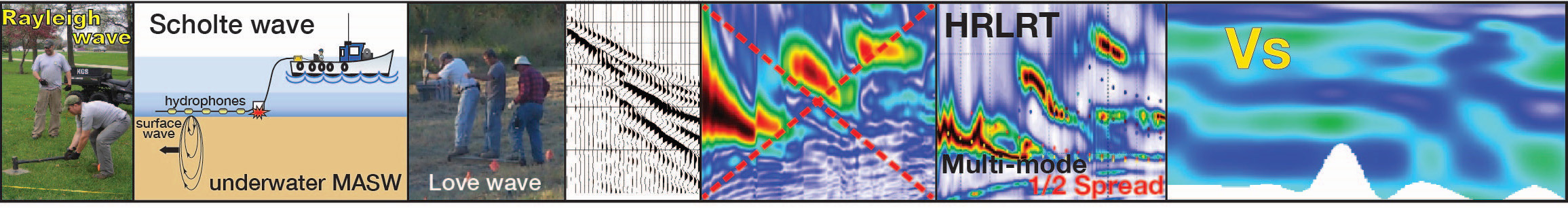
SurfSeis 具有许多独特的功能,自90年代末以来,我们在KGS (MASW的发源地) 专门开发了这些功能,以满足我们对应用研究的需要和解决现实中的问题。
其中的许多功能都是为了满足我们的合作者与软件的合作伙伴的实际需求而开发的 (我们认为SurfSeis 的每个所有者都是我们的合作伙伴)。准确的结果以及各个测站的地下信息准确性一直是我们的主要目标。SurfSeis 是一款成熟的软件 (与1998年首次获得版权) , 其科学,算法和软件的应用程序以及进行了超过十五年的测试,并已通过确认和同行的认可得到了验证。毫无疑问,这在任何地方目前都是最科学,最先进的软件。
据我们了解,许多商业开发软件的工作人员最近开始(最近5年),制作的软件与SurfSeis
类似的灵活性,完整性和准确性,这让我们感到非常兴奋。竞争的最大受益者是我们的用户,并且用户一直是我们关注的焦点,因为我们想将这种不断发展的研究产品提供给所有的人。
我们鼓励商业软件的开发人员尝试与我们的科学,算法效率,应用程序和以用户为中心的功能等想匹配。我们的工作热情来自于我们的研究,但我们正在努力使每个人都能通过SurfSeis尽快获得我们的研究产品。你可以访问以下网页查看我们的发表物(http://www.kgs.ku.edu/software/surfseis/pubs_year_cn.html) 并将其与SurfSeis中的功能进行匹配(详情请参见 http://www.kgs.ku.edu/software/surfseis/index_cn.html网页上的传单) 。 经过出版过程与同行的评审,这些比较与科学的认可可以为您成为 SurfSeis 合作伙伴提供坚实的信心。
以下是SurfSeis 的一些独特功能,它们会在我们的研究中经常被使用,它们可能会对您所从事的许多应用程序有帮助。并且很可能这些功能中的大多数只能在SurfSeis中找到。
1. HRLRT (即., 高分辨率线性radon 变换)
a. 解释基本模态。
b. 解释更高模态。
c. 允许通过使用更短的测线来提高水平分辨率,同时仍然能够遵循频散曲线趋势。
d. 在更高模态下进行转换时,通过使用更多的分层来提高垂直分辨率。
2. 多模态反演
a. 最小化不稳定性。
b. 使用更多没有不稳定性的层来提高垂直分辨率。
c. 增加调查深度。
3. 可变的地形
4. 可变的反演深度
a. 本身可以是一个解释工具。
5. 2D模式查看和导入初始模型功能
a. 帮助避免初始模型错误假设/错误,这种错误无法通过单个一维绘图法检测到。
b. 可以使用Gardner或用户指定的方程参数(即针对特定地点进行调整)从Vp导入密度值或导出2D密度模型。
6. 拉夫波处理
a. 反演不需要Vp的先验信息。
b. 降低了更高模态的可能性,因此基本模态拥有更好的质量。
7. Scholte波 (即, 水下 MASW) 处理
a. 考虑到表面存在水层而不是空气。
8. 瑞利波衰减测量的Qs估计。
9. 窗口分割,以改善被动源数据频散曲线成像。
10. 拼接频散曲线图像。
a. 允许用户从不同的数据通过控制不同的波长进行拼接。此类数据可以使用不同的震源,测线大小或变换,这可以增强最终结果的频散曲线频率范围,水平和垂直分辨率。
具体方法,研究和应用
HRLRT 在使用某些数据时功能非常的强大。在主动数据上,它提供了基本模态的高频率,而常规转换由于更高模态的干扰未能提供这种模态。在某些被动源数据集上,可以查看到一些无法在常规变换中显示的频散曲线图像。此外,如果有多个被动源(例如货运列车)参与 (Ivanov et al., 2013) 则窗口分割可以进一步改善图像。
许多研究人员认为HRLRT优于常规算法 (Zeng et al., 2012; Pan et al., 2013; Yin et al., 2016; Ivanov et al., 2017b; Li et al., 2017)。 如果您发现HRLRT对某些数据集非常有效,那也是在我们意料之中的。
HRLRT 好处, 包括主动和被动源数据Ivanov et al. (2017a)。
HRLRT, 多模态反演, 拉夫波, 和可变深度可在Ivanov et al. (2017c) 找到。
密度趋势使用非恒定的密度值是由我们的Ivanov et al. (2016) 研究的。
Qs 衰减测量的估计是由我们的研究所发动的 (Xia et al., 2002; Xia et al., 2012; Ivanov et al., 2014; Feigenbaum et al., 2016)。获得 Qs 是一个非常具有挑战性的问题,因为很多变量可以导致它产生问题。我们进行了特殊的努力终于使它能顺利运作,其中包括了在二维Vp和密度模型中使用使用特殊的滤噪。据我们所知,SurfSeis是唯一一种提供这种工具的软件。
参考:
Feigenbaum, D., J. Ivanov, R. Miller, S. Peterie, and S. Morton, 2016, Near-surface Qs estimations using multichannel analysis of surface waves (MASW) and the effect of nonfundamental mode energy on Q estimation: An example from Yuma proving ground, Arizona, SEG Technical Program Expanded Abstracts 2016, 4971-4976.
Ivanov, J., B. Leitner, W. T. Shefchik, T. J. Schwenk, and S. L. Peterie, 2013, Evaluating hazards at salt cavern sites using multichannel analysis of surface waves: The Leading Edge, 32, 289-305.
Ivanov, J., R. D. Miller, S. L. Peterie, and G. Tsoflias, 2014, Near-surface Qs and Qp estimations from Rayleigh waves using multi-channel analysis of surface waves (MASW) at an Arctic ice-sheet site, SEG Technical Program Expanded Abstracts 2014, 2006-2012.
Ivanov, J., G. Tsoflias, R. D. Miller, S. Peterie, S. Morton, and J. Xia 2016, Impact of density information on Rayleigh surface wave inversion results: Journal of Applied Geophysics, 135, 43-54.
Ivanov, J., R. Miller, D. Feigenbaum, and J. Schwenk, 2017a, Benefits of using the high-resolution linear Radon transform with the multichannel analysis of surface waves method, SEG Technical Program Expanded Abstracts 2017, 2647-2653.
Ivanov, J., R. D. Miller, D. Feigenbaum, S. L. C. Morton, S. Peterie, and J. B. Dunbar, 2017b, Revisiting levees in southern Texas using Love-wave multichannel analysis of surface waves with the high-resolution linear Radon transform: Interpretation, 5, Accepted for publication.
Ivanov, J., R. D. Miller, D. Feigenbaum, S. L. C. Morton, S. L. Peterie, and J. B. Dunbar, 2017c, Revisiting levees in southern Texas using Love-wave multichannel analysis of surface waves with the high-resolution linear Radon transform: Interpretation, 5, T287-T298.
Li, J., Z. C. Feng, and G. Schuster, 2017, Wave-equation dispersion inversion: Geophysical Journal International, 208, 1567-1578.
Pan, Y. D., J. H. Xia, and C. Zeng, 2013, Verification of correctness of using real part of complex root as Rayleigh-wave phase velocity with synthetic data: Journal of Applied Geophysics, 88, 94-100.
Xia, J. G., Y. X. Xu, R. D. Miller, and J. Ivanov, 2012, Estimation of near-surface quality factors by constrained inversion of Rayleigh-wave attenuation coefficients: Journal of Applied Geophysics, 82, 137-144.
Xia, J. H., R. D. Miller, C. B. Park, and G. Tian, 2002, Determining Q of near-surface materials from Rayleigh waves: Journal of Applied Geophysics, 51, 121-129.
Yin, X., H. Xu, L. Wang, Y. Hu, C. Shen, and S. Sun, 2016, Improving horizontal resolution of high-frequency surface-wave methods using travel-time tomography: Journal of Applied Geophysics, 126, 42-51.
Zeng, C., J. H. Xia, R. D. Miller, G. P. Tsoflias, and Z. J. Wang, 2012, Numerical investigation of MASW applications in presence of surface topography: Journal of Applied Geophysics, 84, 52-60.